Mohr's circle
Mohr's circle, named after Christian Otto Mohr, is a two-dimensional graphical representation of the state of stress at a point. The abscissa,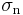 
, and ordinate, 
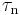, of each point on the circle are the normal stress and shear stress components, respectively, acting on a particular cut plane with a unit vector 
with components 
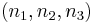. In other words, the circumference of the circle is the locus of points that represent the state of stress on individual planes at all their orientations.
Karl Culmann was the first to conceive a graphical representation for stresses while considering longitudinal and vertical stresses in horizontal beams during bending. Mohr's contribution extended the use of this representation for both two- and three-dimensional stresses and developed a failure criterion based on the stress circle.[1]
Other graphical methods for the representation of the stress state at a point include the Lame's stress ellipsoid and Cauchy's stress quadric.
Contents |
Mohr's circle for two-dimensional stress states
A two-dimensional Mohr's circle can be constructed if we know the normal stresses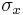 
, 
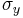, and the shear stress 
. The following sign conventions are usually used:
- Tensile stresses (positive) are to the right.
- Compressive stresses (negative) are to the left.
- Clockwise shear stresses are plotted upward.
- Counterclockwise shear stresses are plotted downward.
The reason for the above sign convention is that, in engineering mechanics[2], the normal stresses are positive if they are outward to the plane of action (tension), and shear stresses are positive if they rotate clockwise about the point in consideration. In geomechanics, i.e. soil mechanics and rock mechanics, however, normal stresses are considered positive when they are inward to the plane of action (compression), and shear stresses are positive if they rotate counterclockwise about the point in consideration.[1][3][4][5]
To construct the Mohr circle of stress for a state of plane stress, or plane strain, first we plot two points in the 
space corresponding to the known stress components on both perpendicular planes, i.e.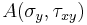 
and 
(Figure 1 and 2). We then connect points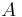 
and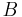 
by a straight line and find the midpoint 
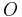which corresponds to the intersection of this line with the 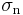axis. Finally, we draw a circle with diameter 
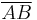and centre at .
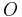The radius 
of the circle is ![R = \sqrt{\left[\tfrac{1}{2}(\sigma_x - \sigma_y)\right]^2 %2B \tau_{xy}^2}\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/1fcdd1b2cf3db0c152af80f8c32fe2b1.png)
, and the coordinates of its centre are ![\left[\tfrac{1}{2}(\sigma_x %2B \sigma_y), 0\right]\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/373f9b807e104e77cd4039ba1b97e609.png)
.
The principal stresses are then the abscissa of the points of intersection of the circle with the 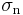axis (note that the shear stresses are zero for the principal stresses).
Drawing a Mohr's circle
The following procedure is used to draw a Mohr's circle and to find the magnitude and direction of maximum stresses from it.
- First, the
- and 
-axes of a Cartesian coordinate system are identified as the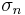 
-axis and 
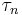-axis, respectively. - Next, two points of the Mohr's circle are plotted. These are the points B (,
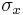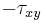) and A (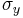, ). The line connecting these two points is a diameter of the Mohr's circle. - The center of the Mohr's circle, O, is located where the diameter, AB, intersects the σ-axis. This point gives the average normal stress (σavg). The average normal stress can be read directly from a plot of the Mohr's circle. Alternatively, it can be calculated using
.
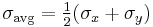- The Mohr's circle intersects the 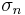axis at two points, C and E. The stresses at these two end points of the horizontal diameter are
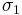and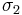 
, the principal stresses. The point 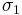represents the maximum normal stress (σmax) and the point 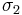is the minimum normal stress (σmin). The equations for finding these values are
![\sigma_1 = \sigma_\max = \tfrac{1}{2}(\sigma_x %2B \sigma_y) %2B \sqrt{\left[\tfrac{1}{2}(\sigma_x- \sigma_y)\right]^2%2B \tau_{xy}^2}](/2012-wikipedia_en_all_nopic_01_2012/I/33e900e386829720ecce7cb89c04a7f8.png)
![\sigma_2 = \sigma_\min = \tfrac{1}{2}(\sigma_x %2B \sigma_y) - \sqrt{\left[\tfrac{1}{2}(\sigma_x- \sigma_y)\right]^2%2B \tau_{xy}^2}](/2012-wikipedia_en_all_nopic_01_2012/I/b3c9288784215dab9733e5c72b2cccd3.png)
- Next we examine the points where the circle intersects the line parallel to 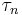-axis passing through the center of the circle, O. The vertical diameter of the circle passes through O (σavg) and goes up to positive
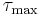and down to negative 
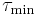. The magnitudes of extreme values are equal to the radius of the Mohr's circle, but with different signs. The equation to find these extreme values of the shear stress is[6]
![\tau_{\max,\min}= \pm \sqrt{\left[\tfrac{1}{2}(\sigma_x- \sigma_y)\right]^2%2B {\tau_{xy}}^2}](/2012-wikipedia_en_all_nopic_01_2012/I/5e19c30e7376a357246f6e515cb53f43.png)
.
- The next value to determine is the angle that the plane of maximum normal stress makes with the -axis. Let us create a new
-axis by drawing a line from the center of the Mohr circle, O, through point A. Let the angle between the -axis and the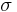 
-axis be 
. If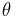 
is the angle between the maximum normal stress and the -axis, then it can be shown that = 2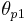
. The angle is found by:
![\phi = 2\theta_{p1} = \arctan\left[2\tau_{xy}/(\sigma_x- \sigma_y)\right]](/2012-wikipedia_en_all_nopic_01_2012/I/424f8cb0061c918a90b5bf7ff418b924.png)
.
- To find the angle that the direction that the plane of maximum shear stress makes with the -axis, we use the relation
![2\theta_s = \arctan\left[-(\sigma_x - \sigma_y)/(2\tau_{xy})\right]](/2012-wikipedia_en_all_nopic_01_2012/I/1541660135d6390a03d01747918a4098.png)
. It is important to pay attention to the use of these two equations as they look similar.
- Often, the final step of the process is to also draw a square stress element indicating the orientations of the maximum normal and shear stresses; the normal stress element at an angle 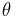and the maximum shear stress element at an angle of
.
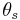The previous discussion assumes, implicitly, that there are two orthogonal directions and that define a plane in which the stress components 
. 
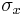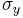, and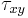 
are known. It is also implicit that these stresses are known at a point 
in a continuum body under plane stress or plane strain. The Mohr circle, once drawn, can be used to find the components of the stress tensor for any other choice of orthogonal directions in the plane.
Stress components on an arbitrary plane
Using the Mohr circle one can find the stress components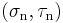 
on any other plane with a different orientation 
that passes through point . For this, two approaches can be used:
- The first approach relies on the fact that the angle between two planes passing through is half the angle between the lines joining their corresponding stress points 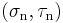on the Mohr circle and the centre of the circle (Figure 1). In other words, the stresses 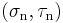acting on a plane at an angle counterclockwise to the plane on which 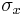acts is determined by traveling counterclockwise around the circle from the known stress point
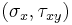a distance subtending an angle 
at the centre of the circle (Figure 1). - The second approach involves the determination of a point on the Mohr circle called the pole or the origin of planes. Any straight line drawn from the pole will intersect the Mohr circle at a point that represents the state of stress on a plane inclined at the same orientation (parallel) in space as that line. Therefore, knowing the stress components
and 
on any particular plane, one can draw a line parallel to that plane through the particular coordinates 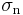and 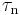on the Mohr circle and find the pole as the intersection of such line with the Mohr circle. As an example, let's assume we have a state of stress with stress components 
, 
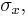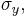, and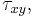 
, as shown on Figure 2. First, we can draw a line from point 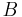parallel to the plane of action of 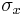, or, if we choose otherwise, a line from point 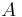parallel to the plane of action of 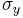. The intersection of any of these two lines with the Mohr circle is the pole. Once the pole has been determined, to find the state of stress on a plane making an angle with the vertical, or in other words a plane having its normal vector forming an angle with the horizontal plane, then we can draw a line from the pole parallel to that plane (See Figure 2). The normal and shear stresses on that plane are then the coordinates of the point of intersection between the line and the Mohr circle.
Mohr's circle for a general three-dimensional state of stresses
To construct the Mohr's circle for a general three-dimensional case of stresses at a point, the values of the principal stresses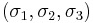 
and their principal directions 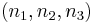must be first evaluated.
Considering the principal axes as the coordinate system, instead of the general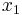 
, 
, 
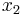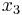coordinate system, and assuming that 
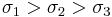, then the normal and shear components of the stress vector 
, for a given plane with unit vector , satisfy the following equations
Knowing that 
, we can solve for 
, 
, 
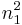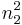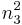, using the Gauss elimination method which yields
Since 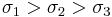, and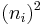 
is non-negative, the numerators from the these equations satisfy
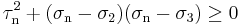as the denominator 
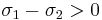and 
as the denominator 
and 
as the denominator 
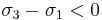and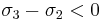 
These expressions can be rewritten as
![\begin{align}
\tau_\mathrm{n}^2 %2B \left[ \sigma_\mathrm{n}- \tfrac{1}{2} (\sigma_2 %2B \sigma_3) \right]^2 \ge \left( \tfrac{1}{2}(\sigma_2 - \sigma_3) \right)^2 \\
\tau_\mathrm{n}^2 %2B \left[ \sigma_\mathrm{n}- \tfrac{1}{2} (\sigma_1 %2B \sigma_3) \right]^2 \le \left( \tfrac{1}{2}(\sigma_1 - \sigma_3) \right)^2 \\
\tau_\mathrm{n}^2 %2B \left[ \sigma_\mathrm{n}- \tfrac{1}{2} (\sigma_1 %2B \sigma_2) \right]^2 \ge \left( \tfrac{1}{2}(\sigma_1 - \sigma_2) \right)^2 \\
\end{align}\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/33821591d920ac61b7b475ef18e44909.png)
which are the equations of the three Mohr's circles for stress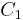 
, 
, and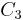 
, with radii 
, 
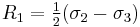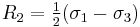, and 
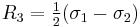, and their centres with coordinates ![\left[\tfrac{1}{2}(\sigma_2 %2B \sigma_3), 0\right]\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/86cb9f6e824b978918a2e484b673d7ea.png)
, ![\left[\tfrac{1}{2}(\sigma_1 %2B \sigma_3), 0\right]\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/c5b8f547ccae7405b3d520dd15577a7e.png)
, ![\left[\tfrac{1}{2}(\sigma_1 %2B \sigma_2), 0\right]\,\!](/2012-wikipedia_en_all_nopic_01_2012/I/35d467ab2ffec0d91fbbbc6a72293990.png)
, respectively.
These equations for the Mohr's circles show that all admissible stress points 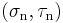lie on these circles or within the shaded area enclosed by them (see Figure 3). Stress points 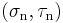satisfying the equation for circle 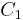lie on, or outside circle 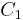. Stress points 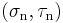satisfying the equation for circle lie on, or inside circle . And finally, stress points 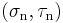satisfying the equation for circle 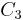lie on, or outside circle .
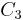References
- ^ a b Parry
- ^ The sign convention differ in disciplines such as mechanical engineering, structural engineering, and geomechanics. The engineering mechanics sign convention is used in this article.
- ^ Jumikis
- ^ Holtz
- ^ Brady
- ^ Megson, T.H.G., Aircraft Structures for Engineering Students, Fourth Edition, 2007, section 1.8
Bibliography
- Beer, Ferdinand Pierre; Elwood Russell Johnston, John T. DeWolf (1992). Mechanics of Materials. McGraw-Hill Professional. ISBN 0-07-112939-1.
- Brady, B.H.G.; E.T. Brown (1993). Rock Mechanics For Underground Mining (Third ed.). Kluwer Academic Publisher. pp. 17–29. ISBN 0-412-47550-2. http://books.google.ca/books?id=s0BaKxL11KsC&lpg=PP1&pg=PA18#v=onepage&q=&f=false.
- Davis, R. O.; Selvadurai. A. P. S. (1996). Elasticity and geomechanics. Cambridge University Press. pp. 16–26. ISBN 0-521-49827-9. http://books.google.ca/books?id=4Z11rZaUn1UC&lpg=PP1&pg=PA16#v=onepage&q=&f=false.
- Holtz, Robert D.; Kovacs, William D. (1981). An introduction to geotechnical engineering. Prentice-Hall civil engineering and engineering mechanics series. Prentice-Hall. ISBN 0-13-484394-0. http://books.google.ca/books?id=yYkYAQAAIAAJ&dq=inauthor:%22William+D.+Kovacs%22&ei=kF-MS5LRKpfCM9vEhIYN&cd=1.
- Jaeger, John Conrad; Cook, N.G.W, & Zimmerman, R.W. (2007). Fundamentals of rock mechanics (Fourth ed.). Wiley-Blackwell. pp. 9–41. ISBN 0-632-05759-9. http://books.google.com/books?id=FqADDkunVNAC&lpg=PP1&pg=PA10#v=onepage&q=&f=false.
- Jumikis, Alfreds R. (1969). Theoretical soil mechanics: with practical applications to soil mechanics and foundation engineering. Van Nostrand Reinhold Co.. ISBN 0-442-04199-3. http://books.google.ca/books?id=NPZRAAAAMAAJ&source=gbs_navlinks_s.
- Parry, Richard Hawley Grey (2004). Mohr circles, stress paths and geotechnics (2 ed.). Taylor & Francis. pp. 1–30. ISBN 0-415-27297-1. http://books.google.ca/books?id=u_rec9uQnLcC&lpg=PP1&dq=mohr%20circles%2C%20sterss%20paths%20and%20geotechnics&pg=PA1#v=onepage&q=&f=false.
- Timoshenko, Stephen P.; James Norman Goodier (1970). Theory of Elasticity (Third ed.). McGraw-Hill International Editions. ISBN 0-07-085805-5.
- Timoshenko, Stephen P. (1983). History of strength of materials: with a brief account of the history of theory of elasticity and theory of structures. Dover Books on Physics. Dover Publications. ISBN 0-486-61187-6.